Alternating polynomial
From Wikipedia, the free encyclopedia
(Redirected from Alternating polynomials)
In algebra, an alternating polynomial is a polynomial  such that if one switches any two of the variables, the polynomial changes sign:
such that if one switches any two of the variables, the polynomial changes sign:
 is said to be alternating in
is said to be alternating in  if it changes sign if one switches any two of the
if it changes sign if one switches any two of the 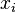 , leaving the
, leaving the  fixed.[1]
fixed.[1]
 such that if one switches any two of the variables, the polynomial changes sign:
such that if one switches any two of the variables, the polynomial changes sign: is said to be alternating in
is said to be alternating in  if it changes sign if one switches any two of the
if it changes sign if one switches any two of the 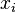 , leaving the
, leaving the  fixed.[1]
fixed.[1]Contents
Relation to symmetric polynomials
Products of symmetric and alternating polynomials (in the same variables ) behave thus:
) behave thus:- the product of two symmetric polynomials is symmetric,
- the product of a symmetric polynomial and an alternating polynomial is alternating, and
- the product of two alternating polynomials is symmetric.
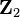 -graded algebra),
where the symmetric polynomials are the even part, and the alternating
polynomials are the odd part. This grading is unrelated to the grading
of polynomials by degree.
-graded algebra),
where the symmetric polynomials are the even part, and the alternating
polynomials are the odd part. This grading is unrelated to the grading
of polynomials by degree.In particular, alternating polynomials form a module over the algebra of symmetric polynomials (the odd part of a superalgebra is a module over the even part); in fact it is a free module of rank 1, with as generator the Vandermonde polynomial in n variables.
If the characteristic of the coefficient ring is 2, there is no difference between the two concepts: the alternating polynomials are precisely the symmetric polynomials.
Vandermonde polynomial
Main article: Vandermonde polynomial
The basic alternating polynomial is the Vandermonde polynomial:The alternating polynomials are exactly the Vandermonde polynomial times a symmetric polynomial:
 where
where  is symmetric. This is because:
is symmetric. This is because: is a factor of every alternating polynomial:
is a factor of every alternating polynomial:  is a factor of every alternating polynomial, as if
is a factor of every alternating polynomial, as if  , the polynomial is zero (since switching them does not change the polynomial, we get
, the polynomial is zero (since switching them does not change the polynomial, we get

- so
 is a factor), and thus
is a factor), and thus  is a factor.
is a factor.
- an alternating polynomial times a symmetric polynomial is an alternating polynomial; thus all multiples of
 are alternating polynomials
are alternating polynomials
Ring structure
Thus, denoting the ring of symmetric polynomials by Λn, the ring of symmetric and alternating polynomials is![\Lambda_n[v_n]](https://upload.wikimedia.org/math/8/3/9/8399bdbd6d0e2d47ef84909efad84c49.png) , or more precisely
, or more precisely ![\Lambda_n[v_n]/\langle v_n^2-\Delta\rangle](https://upload.wikimedia.org/math/9/3/5/9351531687e42167ab372687e32dcdae.png) , where
, where  is a symmetric polynomial, the discriminant.
is a symmetric polynomial, the discriminant.That is, the ring of symmetric and alternating polynomials is a quadratic extension of the ring of symmetric polynomials, where one has adjoined a square root of the discriminant.
Alternatively, it is:
 , and obtains a different relation; see Romagny.
, and obtains a different relation; see Romagny.Representation theory
For more details on this topic, see Representation theory of the symmetric group.
From the perspective of representation theory, the symmetric and alternating polynomials are subrepresentations of the action of the symmetric group on n letters on the polynomial ring in n variables. (Formally, the symmetric group acts on n letters, and thus acts on derived objects, particularly free objects on n letters, such as the ring of polynomials.)The symmetric group has two 1-dimensional representations: the trivial representation and the sign representation. The symmetric polynomials are the trivial representation, and the alternating polynomials are the sign representation. Formally, the scalar span of any symmetric (resp., alternating) polynomial is a trivial (resp., sign) representation of the symmetric group, and multiplying the polynomials tensors the representations.
In characteristic 2, these are not distinct representations, and the analysis is more complicated.
If
 , there are also other subrepresentations of the action of the symmetric group on the ring of polynomials, as discussed in representation theory of the symmetric group.
, there are also other subrepresentations of the action of the symmetric group on the ring of polynomials, as discussed in representation theory of the symmetric group.Unstable
Alternating polynomials are an unstable phenomenon (in the language of stable homotopy theory): the ring of symmetric polynomials in n variables can be obtained from the ring of symmetric polynomials in arbitrarily many variables by evaluating all variables above to zero: symmetric polynomials are thus stable or compatibly defined. However, this is not the case for alternating polynomials, in particular the Vandermonde polynomial.
to zero: symmetric polynomials are thus stable or compatibly defined. However, this is not the case for alternating polynomials, in particular the Vandermonde polynomial.


![R[e_1,\dots,e_n,v_n]/\langle v_n^2-\Delta\rangle.](https://upload.wikimedia.org/math/e/f/5/ef577afa4dc507a71b0f232688e033a2.png)
No comments:
Post a Comment